Alg-tool Quadratic Inequalities

Section 4.4 Quadratic Inequalities

Subsection 1. Solve a linear inequality

First, let's review solving linear inqualities.

Subsubsection Examples

Example 4.46.

Solve \(~-3x+1 \gt 7~\) and graph the solutions on a number line.

\begin{align*} -3x+1 \amp \gt 7 \amp \amp \blert{\text{Subtract 1 from both sides.}}\\ -3x \amp \gt 6 \amp \amp \blert{\text{Divide both sides by -3.}}\\ x \amp \lt -2 \amp \amp \blert{\text{Reverse the direction of the inequality.}} \end{align*}

The graph of the solutions is shown below.

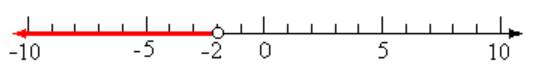
Example 4.47.

Solve \(~-3 \lt 2x-5 \le 6~\) and graph the solutions on a number line.

Solution

\begin{align*} -3 \amp \lt 2x-5 \le 6 \amp \amp \blert{\text{Add 5 on all three sides.}}\\ 2 \amp \lt 2x \le 11 \amp \amp \blert{\text{Divide each side by 2.}}\\ 1 \amp \lt x \le \dfrac{11}{2} \amp \amp \blert{\text{Do not reverse the inequality.}} \end{align*}

The graph of the solutions is shown below.

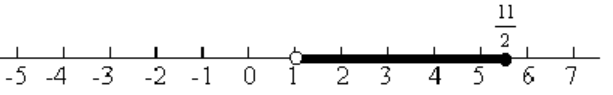
Subsubsection Exercises

Checkpoint 4.48.

Solve the inequality \(~8-4x \gt -2~\)

Answer

\(x \lt \dfrac{5}{2}\)

Checkpoint 4.49.

Solve the inequality \(~-6 \le \dfrac{4-x}{3} \lt 2~\)

Answer

\(22 \ge x \gt -2\)

Checkpoint 4.50.

Solve the inequality \(~3x-5 \lt -6x+7~\)

Answer

\(x \lt \dfrac{4}{3}\)

Checkpoint 4.51.

Solve the inequality \(~-6 \gt 4-5b \gt -21~\)

Answer

\(2 \lt b \lt 5\)

Subsection 2. Find the \(x\)-intercepts of a parabola

To solve a quadratic inequality, we first find the \(x\)-intercepts of the graph. Remember that there are four different methods for solving a quadratic equation.

Subsubsection Examples

Example 4.52.

Find the \(x\)-intercepts of the parabola \(~y=4x^2-12\)

Solution

Set \(y=0\) and solve for \(x\text{.}\) Use extraction of roots.

\begin{align*} 4x^2-12 \amp = 0 \\ 4x^2 \amp = 12 \\ x^2 \amp = 3 \\ x \amp = \pm \sqrt{3} \end{align*}

The \(x\)-intercepts are \((\sqrt{3},0)\) and \((-\sqrt{3},0)\text{,}\) or about \((1.7,0)\) and \((-1.7,0)\text{.}\)

Example 4.53.

Find the \(x\)-intercepts of the parabola \(~y=-4x^2-12x\)

Solution

Set \(y=0\) and solve for \(x\text{.}\) Factor the right side.

\begin{align*} 0 \amp = -4x^2-12x \\ 0 \amp = -4x(x+3) \amp \amp \blert{\text{Set each factor equal to 0.}}\\ 4x\amp = 0~~~~~~x+3 = 0 \\ x \amp = 0~~~~~~x=-3 \end{align*}

The \(x\)-intercepts are \((0,0)\) and \((-3,0)\text{.}\)

Example 4.54.

Find the \(x\)-intercepts of the parabola \(~y=4x^2-12x+8\)

Solution

Set \(y=0\) and solve for \(x\text{.}\) Factor the right side.

\begin{align*} 0 \amp = 4x^2-12x+8 \\ 0 \amp = 4(x^2-3x+2) \\ 0 \amp = 4(x-2)(x-1) \amp \amp \blert{\text{Set each factor equal to 0.}}\\ x-2\amp = 0~~~~~~x-1 = 0 \\ x \amp = 2~~~~~~x=1 \end{align*}

The \(x\)-intercepts are \((2,0)\) and \((1,0)\text{.}\)

Example 4.55.

Find the \(x\)-intercepts of the parabola \(~y=12-12x-4x^2\)

Solution

Set \(y=0\) and solve for \(x\text{.}\) Use the quadratic formula.

\begin{align*} 0 \amp = -4x^2-12x+12 \amp \amp \blert{a=-4,~b=-12,~c=12}\\ x \amp = \dfrac{12 \pm \sqrt{(-12)^2-4(-4)(12)}}{2(-4)}\\ \amp = \dfrac{12 \pm \sqrt{144+96}}{-8}\\ \amp = \dfrac{12 \pm \sqrt{240}}{-8} = \dfrac{12 \pm 4\sqrt{15}}{-8} \amp \amp \blert{\sqrt{240} = \sqrt{16 \cdot 15} = 4\sqrt{15}}\\ \amp =\dfrac{-3 \pm \sqrt{15}}{2} \end{align*}

The \(x\)-intercepts are \(\left(\dfrac{-3 + \sqrt{15}}{2},0\right)\) and \(\left(\dfrac{-3 - \sqrt{15}}{2},0\right)\text{,}\) or about \((0.44,0)\) and \((-3.44,0)\text{.}\)